Moon Composition & Structure
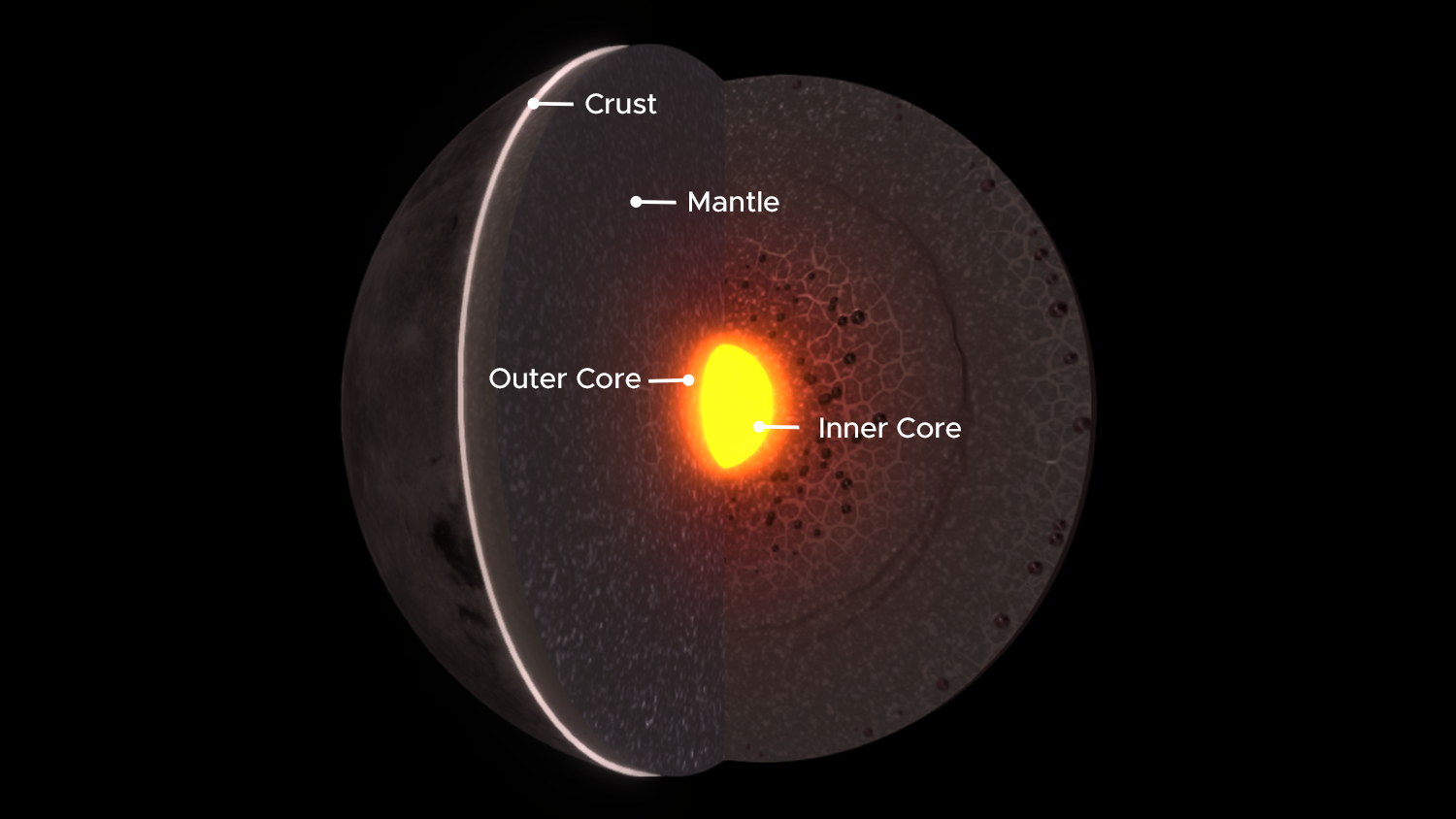
Like Earth, the Moon consists of three main layers: a crust, a mantle, and a core.
Billions of years ago, the Moon was a molten world. As it solidified, the heaviest materials (metals like iron) sank down into the Moon’s center, and the lighter materials (lower density rocks) rose towards the surface. The result is a structure a bit like a chocolate-dipped cherry – solid core, mostly solid/partly liquid mantle, and thin outer crust.
Does the Moon Have an Atmosphere?
Yes, but just barely.
In contrast to Earth’s thick layers of air, the Moon’s exosphere is a bit like the haze you get when you whack a dirty rug with a broom: it includes a sampling of particles knocked free by impact. But instead of a rug, these particles come from the lunar surface. Instead of flying through the air, they’re ejected into near-emptiness. And instead of a broom, the Moon is pummeled by space debris.

Lunar Craters
Earth's Moon is covered in craters.
Lunar craters tell us the history not only of the Moon, but of other worlds, too. On the Moon, where there’s no liquid water or wind, evidence of our solar system's impact history has been preserved for billions of years.

Moon Phases
Earth's view of the half-sunlit Moon shifts throughout the month.
As our perspective changes, we observe a pattern known as lunar phases: new moon, waxing crescent, first quarter, waxing gibbous, full moon, waning gibbous, third quarter, and waning crescent.

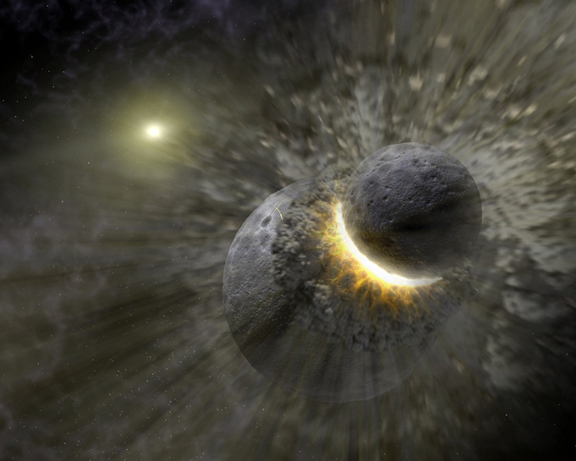
How Did the Moon Form?
Earth’s Moon was born out of destruction.
There are several theories about our Moon’s formation, but almost all share this point in common. Our early solar system was the scene of violent smashups that could end in obliteration or new, larger objects. Those mashed-together objects make up the planets, moons, asteroids and other solar system objects we know today.
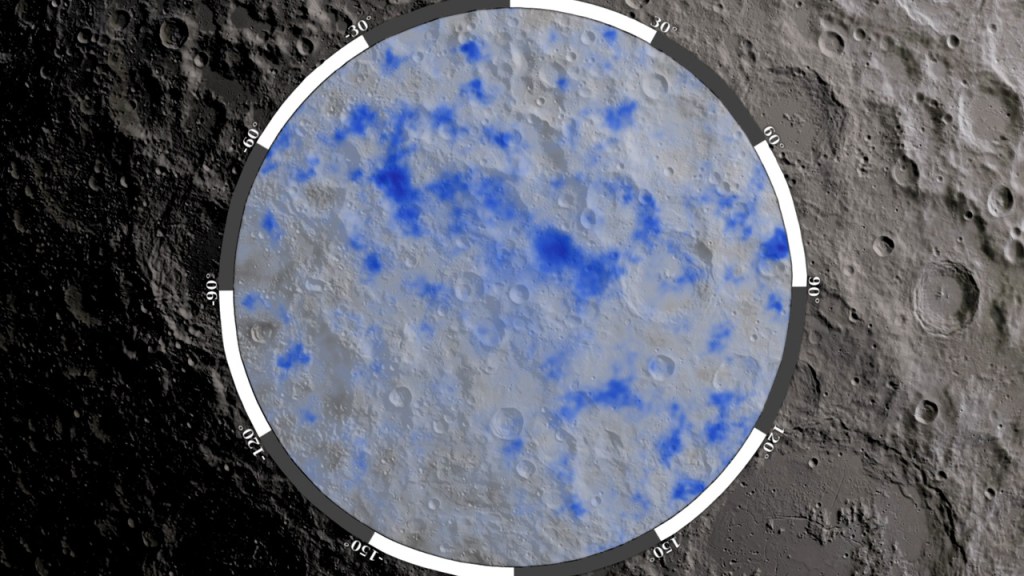
Uncovering Lunar Water
There’s water on the Moon.
Ancient ice hidden deep in permanently shadowed craters. H20 molecules on the sunlit lunar surface. Humanity's search for water on the Moon began hundreds of years ago. Here's a brief history of discoveries leading up to the confirmation of its presence.
Moon Phases 2025
This visualization shows the Moon's phase at hourly intervals throughout 2025, as viewed from the Northern Hemisphere.
About Moon PhasesFeatures
Lunar Reconnaissance Orbiter ImagE:
Far Side of the Moon
The Moon's far side gets as much sunlight as its near side.
Like Earth, the Moon has a day side and a night side, which change as the Moon rotates. The Sun always illuminates half of the Moon while the other half remains dark.
NASA’s Lunar Reconnaissance Orbiter (LRO) mission has mapped the entire lunar surface, including the Moon’s near and far sides, down to a scale of one meter.













































